Computed tomography-based radiostereometric analysis in orthopedic research: practical guidelines

Olof H SANDBERG ¹, Johan KÄRRHOLM ², Henrik OLIVECRONA ³, Stephan M RÖHRL ⁴, Olof G SKÖLDENBERG ⁵, and Cyrus BRODÉN ⁶

¹ Sectra AB, Linköping, Sweden; ² University of Göteborg, Göteborg, Sweden; ³ Department of Molecular Medicine and Surgery, Karolinska Institute, Stockholm, Sweden; ⁴ Division of Orthopaedic Surgery, Oslo University Hospital, Oslo, Norway; ⁵ Department of Clinical Sciences, Unit of Orthopaedics, Karolinska Institutet, Stockholm, Sweden; ⁶ Department of Surgical Sciences, Orthopaedics, Uppsala University Hospital, Sweden

ABSTRACT

Early implant migration is an indicator of the long-term survival/failure of implants. CT-based radio-stereometric analysis (CT-RSA) is a precise method for measuring and visualizing implant migration in vivo using image processing of CT scans. This makes the method widely applicable to orthopedic researcher.

Since its development in the early 2000s, CT-RSA has benefited from breakthroughs in CT and computing technology. These advancements have allowed for the acquisition of images with higher resolution at a much lower radiation dose. As a result, the measurement precision of CT-RSA is now comparable to that of the current gold standard technology while still compatible with most ethical considerations regarding radiation exposure.

In this review we present bests practices for the successful execution of CT-RSA research projects. These practices are based on experience from projects on the hip, knee, shoulder, lower back, cervical spine, foot, pelvis, and wrist.

 

 

Using high-precision measurement methods for implant migration provides an effective means for the early detection of new implant designs with potential poor fixation and inferior survival. This can be achieved through testing in smaller cohorts, effectively limiting the population at risk during the initial phase of clinical introduction [1,2].

The CT-RSA method relies on widely available CT scanners, scanning at low dose. Additionally, it requires no special considerations during surgery, nor does it necessitate modification to the implants or use of 3D templates for the analysis. No metallic bone markers are required, further simplifying the procedure. These advantages make CT-RSA an accessible option for researchers, as it can be performed with minimal specialized equipment or training.

In addition to its primary use for migration studies, the CT-RSA method has been identified as having potential applications in other areas, such as induced displacement CT [3] or for assessing the progression of radiolucent lines [4]. Moreover, the technique holds promise for clinical use, as demonstrated by recent studies [5,6]. Despite using low-dose protocols, most of the CT scans performed have sufficient quality to assess secondary findings, such as bone density measurements [7]. This suggests that the CT-RSA method has the potential to provide more comprehensive information from a single radiation exposure, further increasing its value and usefulness.

Over the past 5 years, we have interacted with a broad range of researchers, both newcomers to migration studies and seasoned experts. We have consistently encountered an expressed desire for a concise, practical guideline. Here we provide practical advice on how to plan, execute, and publish CT-RSA research. The advice was adapted with consideration of the Agree guidelines [8].

Overview of the CT-RSA measurement principle

CT-RSA requires at least 2 subsequent CT-examination volumes. It measures the change in position of one sufficiently radiodense object (bone or implant) relative to another reference object (bone or implant). The main operating principle is illustrated below in Figure 1, using the example of measuring the migration of a tibial component. Note that, for each step, enough documentation on the details of the analysis procedure needs to be created to ensure that if needed the steps can be recreated. A list can be viewed under “documentation and communication of results.” Additionally, any quality control steps performed should also be documented to enable later review. This includes assessment of CT-image quality and visual confirmation of successful merging of reference and moving body. Screenshots and/or written notes can be used to document the quality control process, particularly if the step involves visual components. This will facilitate a more thorough review and aid the accuracy of the research results.

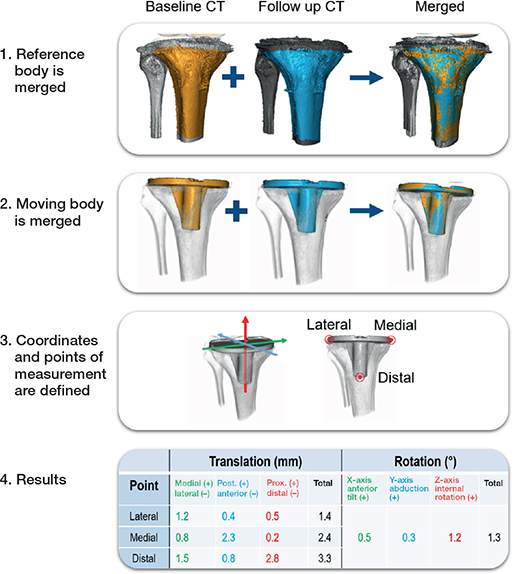
Figure 1. Steps in a CT-RSA measurement.

1. The reference body, typically bone, is identified in the 2 CT images and superimposed via computer-assisted merging. Depending on the software, various methods of quality control will be employed.
2. The moving body, typically a piece of implant, is identified, superimposed, and this is quality controlled.
3. A coordinate system and several measurement points are defined based on anatomical landmarks and/or implant geometry found in the CT.
4. The migration data for the points is calculated. Illustrative images and/or videos can also be created as part of the documentation.

The data is reported as translation in millimeters and rotation in degrees, analogous to current gold standard planar RSA [9]. In clinical papers it is recommended to define the coordinate system using terminology that makes clinical sense to the reader, e.g., distal–proximal, anterior–posterior, abduction–adduction [9]. CT scans acquired sometimes have their coordinate system adjusted according to certain anatomical landmarks already in the radiology department, but regardless it is recommended to fine tune/alter/control these according to the study protocol.

CT-RSA is based on CT and the axis definition in terms of X, Y, Z may differ from the traditional RSA definition. For CT the longitudinal axis is termed Z and the sagittal axis is termed Y and points dorsally (Figure 2). The transverse axis is termed X, the same as for RSA.

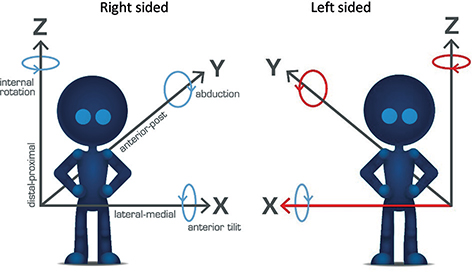
Figure 2. Illustration of migration and rotation definitions for right- and left-sided hip implants with an example of descriptive phrasing for the axis. For a left-sided implant, flip of the positive direction of the rotation around Y and Z and the translation along X makes the results comparable with right-sided implants.

To facilitate interpretation of the results and allow for averaging both right- and left-sided implants the migration data is always translated into the definition of right-sided implants (Figure 2). This means that for left-sided implants translation along the transverse axis (X) and rotation along the longitudinal (Z) and sagittal (Y) axes needs to change sign (be multiplied by –1) to enable comparison between implants on the 2 sides. For the spine, results are typically reported according to the right-sided definition.

CT-RSA may also report total translation (TT) and total rotation (TR). TT is the total distance travelled for a given measurement point regardless of direction. Similarly, TR is the total rotation regardless of direction. Both are calculated using Pythagoras’ theorem. In addition, in RSA there is a measurement called MTPM, or maximum total point motion. This is the largest movement at a given time point of any part of the implant, and it may differ for different time points. A similar measurement to MTPM can be created in CT-RSA corresponding to the highest TT observed.

Preparation of a CT-RSA study project

Check suitability and decide on study details

Table 1 outlines the main steps when planning and conducting a CT-RSA study. It may be that not all research questions are addressable; highly symmetrical implants might hinder measurement of rotational values around the symmetry axis and metal-induced artifacts could, depending on CT settings, have a negative impact on the measurement precision, partly by blurring the implant and/or anchoring bone. Implants made solely of plastic are too radiolucent to show up on a modern CT and currently need metal indicators, such as wire(s) or beads, to be reliably quantifiable in CT-RSA. Different materials can induce varying levels of artifacts in an image, making this variable important to consider. For these reasons, to better understand error sources for a given project, it is recommended to assess a pilot CT of the implant in situ acquired at a radiation dose likely to be used in a study. This may be superfluous if prior CT-RSA studies have already produced an optimal CT protocol.

Table 1. Outline of the main parts of a CT-RSA project

Preparation

1. Check suitability of study idea

2. Decide on study details

3. Adapt and test CT protocol

4. Secure ethical approvals and consider patient data safety if multicenter

Execution

5. Continuous quality control of CT images

6. Ensure safe long-term storage

Evaluation

7. Data production, quality control, and documentation thereof

8. Clinical interpretation of results

9. Documentation and communication of results

The moving and reference bodies need to be defined, as do points of measurement and coordinate system (Figure 2). Choice of time point for CT-scans will vary with research question but common choices for follow-up timepoints are 3 or 6, 12 and 24 months for migration studies and 3, 6, and 12 months for trauma studies. Postoperative CT scans are typically performed within a week after surgery though this may vary depending on study question, availability, and minimization of patient and staff inconvenience.

For adequate interpretation of results, precision estimates are typically needed. Unless precision data has been previously acquired in another study under an identical study protocol this means that precision data needs to be created. Precision data is created from sets of 2 CT scans acquired on the same occasion. This “double examination” is performed in the same way as for standard RSA, i.e., the first CT scan is acquired, the patient is then taken out of the CT scan and placed in a new position whereafter a second CT scan is performed. It is advisable to perform double examinations for at least 20 of the patients in the study. This double exam procedure is described in the RSA ISO standard [10].

Adapt and test CT protocol

To ensure that a proper CT protocol is used, it is recommended to collaborate with a medical physicist who can modify an existing protocol. In some instances, a phantom study may be used to determine a suitable protocol. However, it is important to note that the effective dose may be slightly higher than in the phantom study. Dose considerations are critical, as current radiation recommendations for these types of studies often have an upper limit for the total effective dose per patient of 10 mSv for patients aged above 50 years [11]. As a CT-RSA study can include between 2 and 8 examinations per patient, a dose per examination at no more than 1–2 mSv can be required. For hip, spine, and shoulders, this is typically lower than the standard CT dose for the respective joint. A lower-dose protocol can therefore be needed. This review contains a collection of sample protocols from which to derive inspiration (Table 2). Some general guidelines for adapting a low-dose CT protocol include:

Table 2. Example CT protocols

Source	Region	CT a	Brand	Model	kVp (V)	Exposure (mAs)	Slice thickness (mm)	Increments (mm)	Pitch	Rotation time (s)	Approximate dose (mSv)
Broden et al. 2020	THA	MD	Siemens	Somatom Definition Flash	120	23	0.6	0.6	0.9	1	0.7
	THA	MD	GE	Discovery CT750HD	120	10	0.625	0.312	0.98	1	0.2
Eriksson et al. 2019	THA	MD	Siemens	Somatom Definition Flash	120	23	0.6	0,6	0.9	1	0.6
Cetinic et al. a	Wrist	CB	Planmed	Verity	90	36	0.25	N/A	N/A	N/A	0.002
Angelomenos 2022	THA	MD	GE	Discovery 750 HD	100	Automatic	0.625	0.312	0.984	1	0.8
Engseth et al. b	TKA	MD	GE/Siemens		120	100	0.625	0.625	1.0	0.5	0.07
Poulsen et al. b	Foot	CB	Planmed	Verity	96	64	0.2	N/A	N/A	N/A	0.001
Hansson et al. b	Spine	MD	Siemens	Somatom AS40	140	Automatic (70)	0.6	0.6	0.8	1	0.5
Gerdhem et al. b	Pelvis	MD	Siemens	Somatom Force	100 Sn	Automatic (150)	0.6	0.4	1.2	0.5	0.5
Gerdhem et al. b	Pelvis	MD	Siemens	Somatom Force	100 Sn	Automatic (30)	0.6	0.4	1.2	0.5	0.13
a MD = medical CT, i.e., regular CT. CB = cone beam CT. b Sourced from personal communication (to be published).

• Using approximately half the ampere compared with a normal CT.
• Setting a field of view that includes approximately 5 cm above/below the implant and as many asymmetrical anatomical bone landmarks as possible around the implant.
• Saving CT images with thin (0.6 mm) slices and both with and without metal artifact reduction, at a kernel that also depicts bone well.
• Ensuring that the images are stored long term at full quality and performing quality checks throughout the study to ensure the radiology department adheres to the agreed protocol.
• Activating extended CT scale if available. This increases differentiation of bone and metal by allowing for much increased resolution of differing density levels. The practical consequence is the potential to reduce metal-induced artifacts.

Given that radiation dose is critical, it is important to consider the dose required for each examination in a CT-RSA study. Previous studies have reported doses between 0.2 and 0.7 mSv for a hip examination. This is a large step down from the 3–5 mSv reported for normal-dose hip CT [12]. For comparison, RSA examinations based on conventional radiography have reported a radiation dose for hip at around 0.05–0.15 mSv [13-15]. A given amount of radiation has a lower biological effect in extremities such as knee, wrist, or foot than for example hip, spine, and shoulder. This manifests through a lower conversion factor when calculating the biologically relevant dosing in mSv and the effects are visible in the lower mSv values for the extremities (Table 2).

In many studies practical considerations mean that more than 1 CT machine will be used. This may be due to CT machine replacement or multiple centers involved in the same study. Our experience indicates that CT-RSA is robust to variation in how CTs are used and how the reconstructions are formatted, but that effects on precision may occur. Therefore, studies relying on more than 1 CT should take extra steps to harmonize image settings between the CTs as much as possible. The single most important factor so far seems to be slice thickness, with thicknesses exceeding 1 mm rapidly losing measurement precision compared with a thickness closer to 0.6 mm.

Secure ethical approvals and check patient data safety

If you plan to transfer data from your hospital, for example for a multi-center approach, it is advisable to consult your hospital’s patient data safety professional to understand the necessary requirements. This process varies among hospitals, regions, and countries and could take considerable time; it is therefore best to initiate this step as early as possible.

Most datasets used in orthopedic research carry tags that allow researchers to identify the sample within the research context and potentially identify the patient. This typically makes the term pseudonymized more correct than the term anonymized.

A combination of image acquisition date and time together with a patient-specific ID tag for the study typically enables safe identification while minimizing the patient data footprint.

Execution

Employ continuous quality control of CT images and ensure long-term storage

During the study recruitment and follow-up it is recommended to have continuous quality control as standard image acquisition settings may change over time in one and the same radiology department. Assigning 1 individual to oversee the image acquisition process and ensure that the images conform to the predetermined protocols can help maintain consistency and quality throughout the study. Of note is that most CT machines save the raw data used for the reconstruction for 2 weeks. During those 2 weeks the reconstruction kernel, slice thickness, or metal artifact reduction tools can be adjusted to correct any mistakes. Ensure that no down-sampling of the image quality is done when the images are transferred into long-term storage. If possible, ensure a second point of storage as backup.

Evaluation

Data production and evaluation

Most commonly, migration data at 2-year follow-up is used as the primary endpoint, but for some implant types, e.g., implants fixed without cement, continuous migration between 1 and 2 years might be more clinically relevant. The data can be presented as mean with 95% confidence interval (CI). The interval is created by multiplying the standard deviation (SD) by 1.96. If not found to be normally distributed the data can instead be presented as the median with the interquartile range.

To interpret the clinical relevance of the results, some form of interpretation background is needed. Previous studies have defined acceptable limits for early implant migration based on meta-analyses [16], or solitary implants or implant types [17-19], but for many types of joint prostheses specific documentation is lacking. Different types of migration parameters have been used and the amount of acceptable and clinically relevant difference after 2 years’ observation has varied by up to at least 0.6 mm [20].

Precision measurements should be reported as defined in the RSA ISO standard [10], i.e., by SD multiplied by 1.96. This provides the upper 95% CI of the precision. For cohorts close to or below 20 patients you may prefer to exchange the 1.96 derived from the normal distribution with the T-value. This T-value becomes larger the smaller the sample size the study carries. Using the T-value instead of the 1.96 derived from normal distribution can thus help compensate for the increased uncertainty when working with smaller cohorts, as it provides larger intervals—and therefore indication of uncertainty—for the precision estimates. If the data is not normally distributed the sample mean and SD should instead be reported.

As TT and TR can only be positive, i.e. they are not scattered around 0, hence they need to be specially treated. To determine the precision value of TT and TR, we suggest using the hypotenuse of the precision data for X, Y, and Z, which can be calculated according to the Pythagorean theorem (TT = √(X² + Y² + Z²).

Documentation and communication of results

Video/images can be included in the publication to enhance the visualization of the results and methods. Specimens shown for results should state the rationale behind the choice, for example if it shows the median or illustrates an outlier. Also include:

Study population

• Patient cohort, inclusion and exclusion criteria, and exclusions made.
• The implant type and type of surgical procedure.
• Time points for CT scans.

Image acquisition

• CT protocol; CT brand and model, kV, mAs, slice thickness, kernel, metal artifact reduction y/n, rotation time, increments.
• The average radiation dose per scan and per patient. State the method of calculation.

CT-RSA measurement

• Software used. Include version number and relevant analysis settings.
• Point(s) of measurement.
• Reference body and moving body.
• How the coordinate system was defined.

Data interpretation and statistics

• Exclusions.
• Precision data; state clearly whether sourced from other study or from the same study.
• Power estimation or rationale for choosing the sample size for this study.
• Clinically relevant thresholds.
• Test used, whether data was normally distributed and how this was tested.
• Mean and CI or median and IQR for migration values.

Limitations

CT entails increased radiation exposure compared with conventional RSA. This should be weighed against the added gains. A typical low-dose CT-RSA study could acquire up to 10 hip scans of a given patient and remain below ethical thresholds. While there is no definition in the literature of “low dose” in our experience, sub-1 mSv for hip scans can be one such meaningful definition. Of note is that radiation doses for extremities are so low that, compounded, these are usually 10–100 times lower than typical constraints put on these types of studies. It is worth noting that low-dose CT enables both quantification and clinical assessments, such as bone density measurements [7,21], from the same imaging while conventional planar X-ray RSA requires the addition of CTs for similar study designs.

CT-RSA currently lacks quantifiable quality-control measures similar to what is found in conventional RSA. The suitability of a particular shape to produce high-precision measurements is an example. This number is called the condition number in conventional RSA. Furthermore, there currently is no number indicating the closeness of fit when merging a rigid body from 2 CT scans in CT-RSA, which is called the mean error in conventional RSA. With quantitative measurements lacking, documenting the qualitative impressions of rigid bodies from visual feedback provided by CT-RSA seems to be the second-best option as of now.

Disclosures

OHS is a full-time employee at a company selling a CT-RSA software. All authors are orthopedic surgeons except for OHS, who is an engineer. CB and HO have received remuneration from a company selling CT-RSA software. Completed disclosure forms for this article following the ICMJE template are available on the article page, doi: 10.2340/17453674.2023.15337

1. Hasan S, Marang-van de Mheen P J, Kaptein B L, Nelissen R G H H, Pijls B G. RSA-tested TKA implants on average have lower mean 10-year revision rates than non-RSA-tested designs. Clin Orthop Relat Res 2020; 478(6): 1232-41. doi: 10.1097/CORR.0000000000001209.
2. Nelissen R G H H, Pijls B G, Kärrholm J, Malchau H, Nieuwenhuijse M J, Valstar E R. RSA and registries: the quest for phased introduction of new implants. J Bone Joint Surg Am 2011; 93(Suppl. 3): 62-5. doi: 10.2106/JBJS.K.00907.
3. Wretenberg P, Carlsson S, Tholen S, Olivecrona H. Implant movement analysis (IMA), a new CT based technique for diagnosis of aseptic loosening of total knee arthroplasty. Orthop Res Online J 2021; 8(3): 868-72. doi: 10.31031/OPROJ.2021.08.000690.
4. Brodén C, Reilly P, Khanna M, Popat R, Olivecrona H, Griffiths D, et al. CT-based micromotion analysis method can assess early implant migration and development of radiolucent lines in cemented glenoid components: a clinical feasibility study. Acta Orthop 2022; 93: 277-83. doi: 10.2340/17453674.2022.1976.
5. Berger R, Fletcher F, Donaldson T, Wasielewski R, Peterson M, Rubash H. Dynamic test to diagnose loose uncemented femoral total hip components. Clin Orthop Relat Res 1996; (330): 115-23. doi: 10.1097/00003086-199609000-00014.
6. Sandberg O, Carlsson S, Harbom E, Cappelen V, Tholén S, Olivecrona H, et al. Inducible displacement CT increases the diagnostic accuracy of aseptic loosening in primary total hip arthroplasty. Acta Orthop 2022; 93: 831-6. doi: 10.2340/17453674.2022.5240.
7. Stigbrand H, Brown K, Olivecrona H, Ullmark G. Implant migration and bone mineral density measured simultaneously by low-dose CT scans: a 2-year study on 17 acetabular revisions with impaction bone grafting. Acta Orthop 2020; 91(5): 571-5. doi: 10.1080/17453674.2020.1769295.
8. Brouwers M C, Kerkvliet K, Spithoff K; AGREE Next Steps Consortium. The AGREE Reporting Checklist: a tool to improve reporting of clinical practice guidelines. BMJ 2016;3 52: i1152. doi: 10.1136/bmj.i1152.
9. Valstar E R, Gill R, Ryd L, Flivik G, Börlin N, Kärrholm J. Guidelines for standardization of radiostereometry (RSA) of implants. Acta Orthop 2005; 76(4): 563-72. doi: 10.1080/17453670510041574.
10. ISO SIoIS. ISO 16087:2013 Implants for surgery: Roentegen stereophotogrammetric analysis for the assessment of migration of orthopaedic implants. Geneva: ISO.
11. European Commission. Radiation Protection 99. Guidance on medical exposures in medical and biomedical research; 1998. Available from: https://energy.ec.europa.eu/system/files/2014-11/099_en_1.pdf.
12. Geijer M, Rundgren G, Weber L, Flivik G. Effective dose in low-dose CT compared with radiography for templating of total hip arthroplasty. Acta Radiol 2017; 58(10): 1276-82. doi: 10.1177/0284185117693462.
13. Valstar E R, De Jong F W, Vrooman H A, Rozing P M, Reiber J H C. Model-based Roentgen stereophotogrammetry of orthopaedic implants. J Biomech 2001; 34(6): 715-22. doi: 10.1016/s0021-9290(01)00028-8.
14. Blom I F, Koster L A, Brinke B Ten, Mathijssen N M C. Effective radiation dose in radiostereometric analysis of the hip. Acta Orthop 2020; 91(4): 390-5. doi: 10.1080/17453674.2020.1767443.
15. Brodén C, Sandberg O, Olivecrona H, Emery R, Sköldenberg O. Precision of CT-based micromotion analysis is comparable to radiostereometry for early migration measurements in cemented acetabular cups. Acta Orthop 2021; 92(4): 419-23. doi: 10.1080/17453674.2021.1906082.
16. Pijls B G, Nieuwenhuijse M J, Fiocco M, Plevier J W, Middeldorp S, Nelissen R G, et al. Early proximal migration of cups is associated with late revision in THA. Acta Orthop 2012; 83(6): 583-91. doi: 10.3109/17453674.2012.745353.
17. Kärrholm J, Borssén B, Löwenhielm G, Snorrason F. Does early micromotion of femoral stem prostheses matter? 4–7-year stereoradiographic follow-up of 84 cemented prostheses. J Bone Joint Surg Br 1994;76(6): 912-17.
18. Ryd L, Albrektsson B E, Carlsson L, Dansgard F, Herberts P, Lindstrand A, et al. Roentgen stereophotogrammetric analysis as a predictor of mechanical loosening of knee prostheses. J Bone Joint Surg Br 1995; 77(3): 377-83.
19. Gudnason A, Adalberth G, Nilsson K-G, Hailer N P. Tibial component rotation around the transverse axis measured by radiostereometry predicts aseptic loosening better than maximal total point motion. Acta Orthop 2017; 88(3): 282-7. doi: 10.1080/17453674.2017.1297001.
20. Dyreborg K, Andersen M R, Winther N, Solgaard S, Flivik G, Petersen M M. Migration of the uncemented Echo Bi-Metric and Bi-Metric THA stems: a randomized controlled RSA study involving 62 patients with 24-month follow-up. Acta Orthop 2020; 91(6): 693-8. doi: 10.1080/17453674.2020.1802682.
21. Eriksson T, Maguire G Q, Noz M E, Zeleznik M P, Olivecrona H, Shalabi A, et al. Are low-dose CT scans a satisfactory substitute for stereoradiographs for migration studies? A preclinical test of low-dose CT scanning protocols and their application in a pilot patient. Acta Radiol 2019; 60(12): 1643-52. doi: 10.1177/0284185119844166.